Remainders, in a blog about fractions? Here's a miscellaneous collection of some of the many tasks for which there is not room to develop a weekly set. You might want to develop some variants yourself...
Task 20A: This looks like a standard equivalent fractions task, but it might provoke a short pause for thought. There is not a whole number multiplier that maps the numerator 3 onto the numerator 4, and that could then have been used to map the denominator 60 onto the missing denominator. However the task can be solved easily enough by using the whole number multiplier ×20 to map the numerator 4 onto the missing denominator.
We could of course use the fractional multiplier ×1⅓ to map 3 onto 4 and 60 onto the unknown denominator. Or we could divide the numerator and denominator by 3 to get the fraction 1/20 and then multiply the new numerator and denominator by 4.
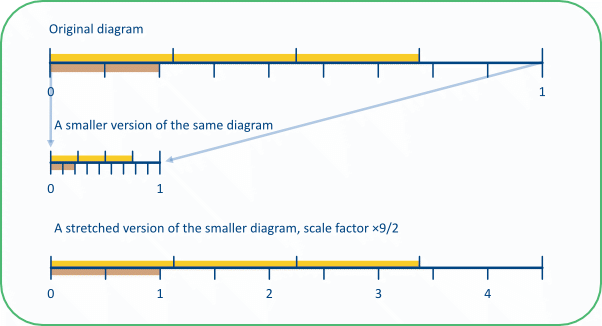
Task 20B: In the first item, the fraction 4/45 is one third of 4/15, so its position is 1 cm from the left end of the rod. Some pupils might hastily think that 4/45 is three times as big as 4/15. Others might be thrown by the fact that the rod is not a whole number of centimeters long and that its length does not seem to have an obvious relationship to any of the other numbers that occur in the item. The faction 4/5 in the second item is more familiar than 4/45 so pupils should have a better idea of where it lies on the rod, namely quite close to the right end. In fact, being 3 times as large as 4/15, it lies at a mark 9 cm along the rod.
A variant of the task would be to ask pupils to find the position and value of the fraction given by
• 4/15 ÷ 3
• 4/15 × 3.
Would pupils find this more or less challenging?
The rod looks to be about 11.2 cm long. It might be interesting to ask pupils what the precise length should be if 4/15 of the rod is 3 cm long.
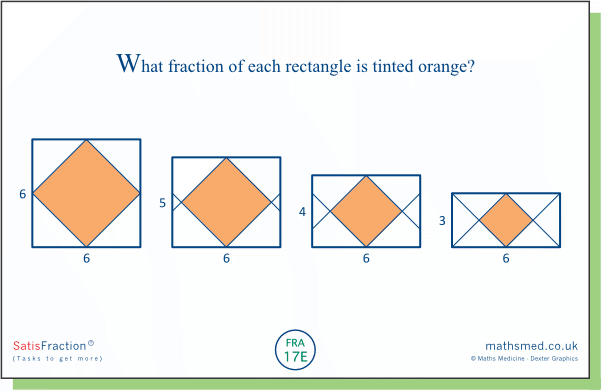
Task 20C: Here it is obvious that ¼ of Bar A is tinted yellow. Some pupils might see immediately that this is also true for Bar B. Others might get there after first determining that 3/12 of the bar is yellow. It turns out that Bar C is also a quarter yellow, even though we cannot assess the exact size of two of the yellow regions.
We can think of Bar C as being split into three regions, each of which is ¼ yellow. This means ¼ of the whole bar is tinted yellow.
We can think of this as an example of the distributive law:
¼ of X + ¼ of Y + ¼ of Z = ¼ of (X + Y + Z).
Task 20D: This is quite a simple task. The nice thing about it is that it can be solved in many different ways. For example, we could split the tinted shape into two triangles (with bases of 2 and 3 units and heights of 5 units, where the whole shape is a 5 by 5 square); or we could apply an area-of-trapezium formula to the existing shape; or we could shear the trapezium so that two corners are right-angled, and we could then cut-and-rejoin the shape to produce a 2½ units by 5 units rectangle.
Note that the task would still work if the whole shape were a rectangle or parallelogram rather than a square.
The variants, below, of the given shape are all half green. We can derive the fraction from the lengths of the parallel sides, namely (2+3)/(5+5).
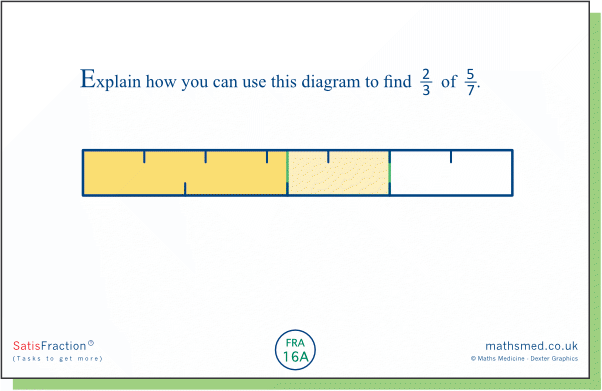
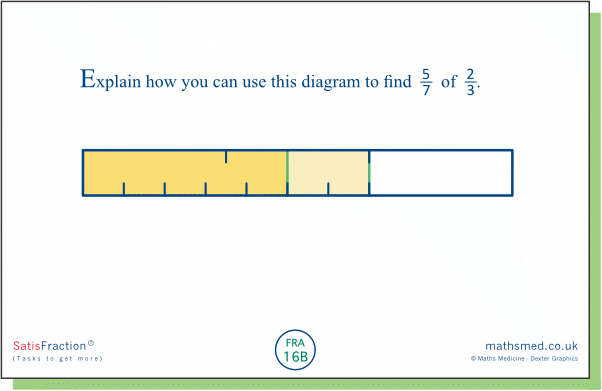
Task 20E: This task can be solved in many different ways and at different levels of abstraction. Some pupils might count small squares: there are 15 tinted yellow out of a total of 60 small squares, so the desired fraction is 15/60. Others might derive these numbers by multiplying: there are 3×5 small yellow squares out of a total of 10×6 small squares, so the fraction is 3×5/10×6 = 15/60 = 1/4.
Others might work more abstractly (or maybe just more procedurally?) by multiplying the given fractions ⅚ and ³⁄₁₀. Note that this essentially gives us the same expression as 3×5/10×6, above, even though the thinking might be very different! For example, some pupils who multiply the fractions might be thinking in terms of scaling: we can transform the large blue rectangle into the yellow rectangle by scaling its height by the scale factor ⅚ and then scaling its width by the scale factor ³⁄₁₀. Or, pupils might adopt a similar-looking but more common static approach: we can form the yellow part of the large rectangle by first taking a ⅚ part of the rectangle and then a ³⁄₁₀ part of that: ³⁄₁₀ of ⅚ of 1 = 1/4.
The product 3/10 of 5/6, or 15/60, reduces so neatly to ¼ because 3 is a factor of 6 and 5 is a factor of 10. We can illustrate this by rotating the tinted region through 90˚, as shown below. We can see that the fraction is a quarter.
The above rotation method works so neatly because of the way the task has been contrived. However, even where such a rotation does not produce an easily recognisable fraction, it nicely illustrates that a product a/u × b/v is equivalent to b/u × a/v. When u = v = 1, this is the same phenomenon as when we rotate an array showing the product of whole numbers a × b to demonstrate that a × b = b × a.
Task 20F: We finish with a method for performing division that appeared in the book Liber Abaci (or the Book of Calculations) written by Leonardo of Pisa (also known as Fibonacci). The book first appeared in 1202, followed by a second version in 1228.
According to Sigler (2002),‘Leonardo’s stated intention was to introduce the Hindu number system and its operations to the Italian people’. The book had a huge influence on the western world.
Sigler, L. E. (2002). Fibonacci’s Liber Abaci. Leonardo Pisano’s Book of Calculation. Springer-Verlag New York.
It is an interesting challenge to show why the method works and to decipher the strange looking ‘fraction’.
It turns out that 1 2 4 / 3 5 5 represents 1/3×5×5 + 2/5×5 + 4/5. Once that has been determined, it is relatively easy to show that this is equivalent to 67/75.