This Week's tasks involve the use of benchmarks, which provides a powerful way to estimate the size of fractions and to compare them. However, Clarke and Roche's study suggests that there are many pupils who don't spontaneously use them - and so should be helped to do so!
TASK 02A: Pupils might find this task to be very challenging, unless they are attuned to, or happen to hit upon, the use of benchmark fractions. If pupils notice that 4/15 is close to a quarter (it is slightly greater), then the task can become very easy: 11/83 lies between 1/7 and 1/8 and so is much smaller.
TASK 02A: This item was used by Clarke and Roche. It turned out to be relatively easy - the third easiest of their 8 items, with 59% of their 11-12 year olds choosing 5/8 with a valid reason. Notice, however, that the benchmark fraction 1/2 is given explicitly. The outcome was very different when the benchmark was implicit, as we discuss below.
TASK 02C: Here is a task that could be used to promote the idea of benchmarks by having fractions where one benchmark is explicit (1/3) and where a second (1/4) is relatively easy to construe.
Of course, the item can still be solved relatively easily in other ways - for example by converting 1/3 to 19/57 so that the fractions have common numerators, or by converting them to fractions with numerators 1×80=80 and 3×19=57 and common denominator 3×80.
It is quite a nice challenge (for us, but perhaps also for pupils) to devise variants of the above task where such alternative strategies become less likely (or, if one wishes, more likely).
For example, though the item below has been made more demanding, in that is slightly less obvious that the second fraction is close to 1/4, it can still be solved as easily as before using common numerators and even more easily using common denominators if pupils happen to see that 81 is a multiple of 3.
On the other hand, the use of common numerators or common denominators are both more demanding here.
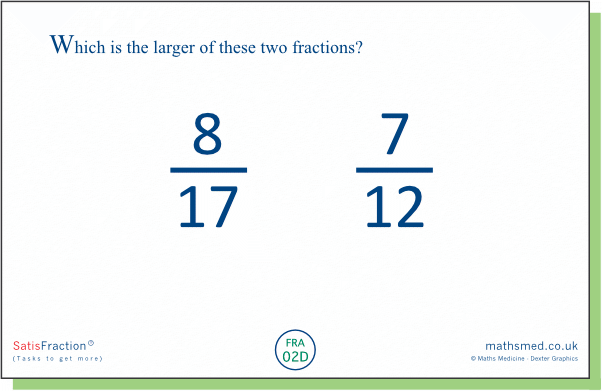
TASK 02D: This pair of fractions was used in a study by Lisa Fazio and colleagues in the United States. It is an interesting study, but it involved adults rather than school pupils, and the samples were rather small (19 undergraduates at a highly selective university on an introductory psychology course, and 19 students at a nonselective community college on an introductory psychology or history course).
The study used a battery of 48 fraction items, six of which, including the above item, were classed as 'Halves Referencing'; these could be solved by noticing that the fractions lie either side of 1/2.
Both samples of students were fairly successful on this group of items, with an average success rate of 93% for the selective students and 73% for the nonselective students. [Responses were classed as 'successful' if the correct fraction was chosen regardless of the nature of any explanation.]
Given that the fractions could be solved using 1/2 as a benchmark, it is interesting to note that only just over half (61%) of the explanations given by the selective students involved this method; the next most common explanations involved common denominators (9%) and converting the fractions to decimals (6%). For the nonselective students, the corresponding percentages were just 13%, and 5% and 3%. This suggests that if we want pupils to benefit from the use of fractions as benchmarks we might need to actively encourage them to do so! This, and the other of Fazio's 'Halves Referencing' tasks, might be good tasks for doing so.
TASK 02E: This is another item used by Fazio and colleagues. They didn't classify it as 'Halves Referencing' since one of the fractions is much closer to 0 than a half, but it was still quite commonly solved by using 1/2 as a benchmark, as in this archetypal explanation:
9/19 is closer to 1/2 and 2/17 is closer to 0 so 9/19 is larger.
Fazio et al also report that some students gave more informal, more qualitative, explanations along these lines:
Since the denominators are close together, we can look at the numerators to see which fraction is larger. 9/19 has a larger numerator so it is larger than 2/17.
Such an explanation isn't watertight, but it shows nice fraction sense nonetheless.
When Laurie Jacques and I used the item with young pupils we came across an interesting and fundamental difficulty; as we discussed whether 9/19 was close to a half, some pupils who had the nice idea of expressing 1/2 in terms of 19ths (which would give 9½/19), struggled to divide 19 by 2 because 19 is odd.