This Week's tasks are fairly straightforward. We measure quantities (mostly lengths) which don't consist of just a whole number of units - in other words, we need to make use of fractional parts of the unit. This is a nice, grounded way of bringing fractions into play, and one that emphasises the notion of fraction as measure or number in quite a simple way.
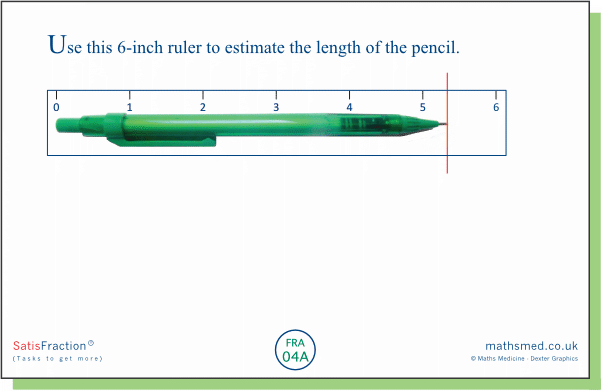
Task 04A: The key idea here is that the length of the (mechanical) pencil is 'five and a bit' inches, and that it would be nice to have a numerical way of describing the 'bit'. This idea won't be new to most pupils but it can be helpful to meet it again!
Some pupils might say the pencil is 'slightly less than 5½ inches'. Other might suggest it could be 5¼ inches or 5⅓. A few(?) might even suggest a mixed number involving a non-unit fraction such as 5⅖.
You might want to ask pupils how they decided on their answer. Did they imagine partitioning the interval from 5 to 6 into equal parts, or did they compare the 'bit' to various familiar benchmark fractions (in particular ½, but perhaps also ¼ and/or ⅓)? Or did they consider what proportion of the 5 to 6 interval was covered by the 'bit'? It turns out that the length is 5⅓ inches, as we confirm in the next task.
Task 04B: Here we measure with a variety of rulers, where the inches have been partitioned in some of the ways that were common on school rulers before decimalisation in the 1970s: quarters, tenths, twelfths (sixteenths were also common). None of these rulers confirm that the pencils' length is actually 5⅓ inches, but they could lead to productive discussions about whether the pupils' earlier estimates might be valid.
The last ruler actually shows 5⁴⁄₁₂. Do pupils recognise that this is the same as 5⅓?
Task 04C: A fairly simple task. Pupils can probably see that the blue line is longer: it is nearer to the '3'. But can they justify it?
Alternatively one could focus on the 'bits' themselves: How can we explain why is 8/10 larger than 3/4?
This version of the task (below) might help pupils see the lengths and the partitions more clearly:
Task 04D: Another fairly simple 'measurement' task. It is easy to see that the orange bar is longer, but can pupils use fractions to explain why?
Or we could consider the benchmark ½ (or 2½), by comparing 2/10 and 4/12 (which is 2/6).
Again, this version (below) might help pupils see things more clearly:
Task 04E: Here the context is time rather than length. The key idea is that the amount of time (the time the train takes from London to Liverpool) is '2 and a bit hours' where the 'bit' is slightly less than ½.
It is difficult to tell whether the time that Jack's train took is equal to, less, or more that 2⅓ hours. But do pupils appreciate that the time taken is 2 hours, plus a 'bit' that we can represent as a fraction of an hour, and that this fraction is less than ½?
In this version of the task, we can see that the time taken is 2⅖ hours (as far as we can tell by eye). Is ⅖ equal to, less than, or more than ⅓?